FACARD 2019 WORKSHOP
FACARD 2019 WORKSHOP
Dates
16 – 18 January 2019
Early-bird registration deadline
30 November 2018
Location
Room T1 on the second floor of the Faculty of Mathematics
IMUB, Barcelona
Commutative algebra is the branch of pure mathematics which studies commutative rings, and their ideals and modules. Many fundamental problems and questions in commutative algebra originated from the neighbouring fields of algebraic geometry and algebraic number theory, and it still benefits from the mutual interactions with them. This is particularly evident when one studies rings of positive characteristic. For example, the geometric theory of vector bundles and their relations with the Frobenius homomorphism has been successfully applied to tight closure and Hilbert-Kunz problems in commutative algebra. More recently, the theory of perfectoid spaces developed by Peter Scholze in the context of arithmetic geometry has been used to tackle The Homological Conjectures in the case of mixed characteristic.
The focus of the workshop is on these recent developments and other related topics. It is addressed to Ph.D. students, postdocs and interested researchers in general. There will be two mini-courses at graduate level and research talks given by international experts and young emerging scientists.
You may find all the details about the programme, registration, social events and updated news on the web of the Conference.
Registration:
Please find all the info about registration here.
Programme of the courses
Asymptotic properties of differential operators around a singularity
Holger Brenner (Universität Osnabrück)
For a local algebra R over a field, we study the decomposition of the module of principal parts. A free summand of the n-th module of principal parts is essentially the same as a differential operator E of order greater or equal than n with the property that the partial differential equation E(f) =1 has a solution. The asymptotic behavior of the size of the free part gives a measure for the singularity represented by R. We compute this invariant, called the differential signature, for invariant rings, toric monoid rings, determinantal rings, quadrics, and we compare it with the F-signature, which is an invariant in positive characteristic defined by looking at the asymptotic decomposition of the Frobenius. This is joint work with Jack Jeffries and Luis Nuñez Betancourt.
Homological conjectures/theorems in commutative algebra
Linquan Ma (Purdue University)
The homological conjectures have been a focus of research in commutative algebra since 1960s. They concern a number of interrelated conjectures concerning various homological properties of commutative rings to their internal ring structures. These conjectures had largely been resolved for rings that contain a field, but several remained open in mixed characteristic—until recently Yves Andre proved Hochster’s direct summand conjecture, which lie in the heart of the homological conjectures. This lecture will focus on the recent breakthroughs. In particular, we will talk about the direct summand conjecture and the existence of big Cohen-Macaulay algebras in positive and mixed characteristic. The main new ingredient in the solution in the mixed characteristic case is the use of perfectoid spaces, and we will give a brief introduction to this subject. We will also discuss some interesting open questions.
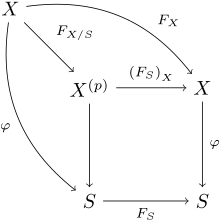
Dates
16 – 18 January 2019
Deadline
30 November 2018
Location
Room T1 on the second floor of the Faculty of Mathematics
IMUB, Barcelona

Commutative algebra is the branch of pure mathematics which studies commutative rings, and their ideals and modules. Many fundamental problems and questions in commutative algebra originated from the neighbouring fields of algebraic geometry and algebraic number theory, and it still benefits from the mutual interactions with them. This is particularly evident when one studies rings of positive characteristic. For example, the geometric theory of vector bundles and their relations with the Frobenius homomorphism has been successfully applied to tight closure and Hilbert-Kunz problems in commutative algebra. More recently, the theory of perfectoid spaces developed by Peter Scholze in the context of arithmetic geometry has been used to tackle The Homological Conjectures in the case of mixed characteristic.
The focus of the workshop is on these recent developments and other related topics. It is addressed to Ph.D. students, postdocs and interested researchers in general. There will be two mini-courses at graduate level and research talks given by international experts and young emerging scientists.
You may find all the details about the programme, registration, social events and updated news on the web of the Conference.
The organizers are BGSMath postdoctoral Fellow Alessio Caminata and BGSMath Faculty member Santiago Zarzuela Armengou.
Registration:
Please find all the info about registration here.
Programme of the courses
Asymptotic properties of differential operators around a singularity
Holger Brenner (Universität Osnabrück)
For a local algebra R over a field, we study the decomposition of the module of principal parts. A free summand of the n-th module of principal parts is essentially the same as a differential operator E of order greater or equal than n with the property that the partial differential equation E(f) =1 has a solution. The asymptotic behavior of the size of the free part gives a measure for the singularity represented by R. We compute this invariant, called the differential signature, for invariant rings, toric monoid rings, determinantal rings, quadrics, and we compare it with the F-signature, which is an invariant in positive characteristic defined by looking at the asymptotic decomposition of the Frobenius. This is joint work with Jack Jeffries and Luis Nuñez Betancourt.
Homological conjectures/theorems in commutative algebra
Linquan Ma (Purdue University)
The homological conjectures have been a focus of research in commutative algebra since 1960s. They concern a number of interrelated conjectures concerning various homological properties of commutative rings to their internal ring structures. These conjectures had largely been resolved for rings that contain a field, but several remained open in mixed characteristic—until recently Yves Andre proved Hochster’s direct summand conjecture, which lie in the heart of the homological conjectures. This lecture will focus on the recent breakthroughs. In particular, we will talk about the direct summand conjecture and the existence of big Cohen-Macaulay algebras in positive and mixed characteristic. The main new ingredient in the solution in the mixed characteristic case is the use of perfectoid spaces, and we will give a brief introduction to this subject. We will also discuss some interesting open questions.
BGSMath Organisers

Holger Brenner (Universität Osnabrück)
Biosketch

Linquan Ma (Purdue University)
